If we have 2 curves y_2 and y_1 that enclose some area and we rotate that area around the x-axis then the volume of the solid formed is given by. Find the volume generated by rotating about x-axis the region in the xy- plane bounded below and above respectively by the curves yx2and yx and sketch the.
Volume Of Rotation Cylindrical Shells About The X Axis Or Y Example 2 Volume Calculus Axis
So lets think about what happens when we rotate this thing around the x-axis.

. Formula is used to find the volume obtained when the area under the graph is rotated by the x axis or y axis Formula must be in x and have y as its subject in order to be rotated about the x axis and the limits are points on the x axis Example. Find the volume in cubic units generated by rotating a circle x2 y2 6x 4y 12 0 about the y-axis. We have a input function y fx and we have to find the volume along x-axis and y-axis.
Enter in the function in the blue input box below. Solids of Revolutions - Volume Added Apr 30 2016 by dannymntya in Mathematics Calculate volumes of revolved solid between the curves the limits and the axis of rotation. So x could be equal to 0 or x minus 3 is equal to 0.
So this is going to stretch our powers of visualization here. 6- 5- 4- Find the volume of the solid created by rotating the following curve y 3x about the y-axis. Enter the exact answer or round it to three decimal places.
To the right is displayed what the solid of revolution would look like if you rotated the displayed area about the x-axis. 1 Recall finding the area under a curve. The volume generated by the circle that revolved about the y-axis is 5922 cubic units.
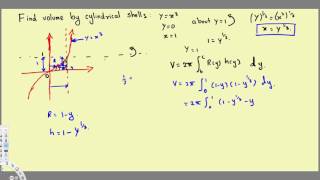
3- 2- Question Transcribed Image Text. 5- 4- Find the volume of the solid created by rotating the following curve y 3vx about the y-axis. V π dc f y 2 dy.
Find the volume of the region rotated around the x-axis formed by f x sec2x vtanx y 0 and x. Volume obtained when rotated 360 by axis Formula. Volume by Rotating the Area Enclosed Between 2 Curves.
Your first 5 questions are on us. X 0 and x - NOTE. So x is equal to 0 or x is equal to 3.
Finding the volume is much like finding the area but with an added component of rotating the area around a line of symmetry usually the x or y axis. In the next video well actually evaluate this integral. Now were going to apply that same idea not to find the area under this curve but to find the volume if we were to rotate this curve around the x-axis.
Adjust the a and b values by using either the sliders or entering them in the input boxes yourself. My Applications of Integrals course. The volume of the solid formed by revolving the region bounded by the curve x f y and the y-axis between y c and y d about the y-axis is given by.
Volumepi int_aby_22-y_12dx In the following general graph y_2 is above y_1. Finding the volume of a solid revolution is a method of calculating the volume of a 3D object formed by a rotated area of a 2D space. Yfrac4x24 from x0 to x2.
Shell Method Around X Axis - 15 images - washer method calculate volume bruin blog volume by washer method around the y axis youtube answered use the shell method to find the volume bartleby solved use the shell method to find the volume of the sol. Were going to go from x equals 0 to x equals 3 to get our volume. Get step-by-step solutions from expert tutors as fast as 15-30 minutes.
Find the volume of the region bounded by y cos x y 0 37 rotated around the x-axis. Solid of revolution The minimum and maximum values of x are given. Example 1 Determine the volume of the solid obtained by rotating the region bounded by y x2 - 4x 5 x 1 x 4 and the x-axis about the x-axis.
So that gives us our interval. So this is x is 0 and this right over here is x is equal to 3. We will calculate this volume by summing discs of the width dx that each have an area of pi y2 where.
Find volume of solid of revolution step-by-step. Show Solution The first thing to do is get a sketch of the bounding region and the solid obtained by rotating the region about the x-axis. Find the volume of the region rotated around the x-axis formed by f x sec2x vtanx y 0 and x.
Solid of Revolution Finding Volume by Rotation. Calculus questions and answers. Answer 1 of 2.

Using The Method Of Cylindrical Shells Find The Volume Of The Solid Obta Method Volume Calculus

Find The Volume Of The Solid Obtained By Rotating The Region Bounded By Volume Calculus Region

Ex 2 Volume Of Revolution Using The Disk Method Sine Squared Function Revolution Method Calculus

0 Comments